geometric of geometric series equation

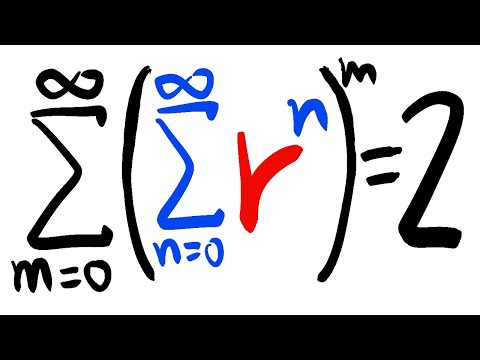
This is a double geometric series equation that I came up with for my precalculus students and calculus 2 students. We are not evaluating the sum of an infinite geometric series, but rather, we are solving for the common ratio to make this equation true. But does it have any solution? Hint, we have to pay attention to the interval of convergence of a power series of a function. Subscribe for more precalculus & calculus tutorials 👉 https://bit.ly/just_calc

The canvas print in the video: All series convergence tests: 👉 https://bit.ly/3GW8EJI
Use "WELCOME10" for 10% off
---------------------------------------------------------
If you find this channel helpful and want to support it, then you can
join the channel membership and have your name in the video descriptions:
👉https://bit.ly/joinjustcalculus
buy a math shirt or a hoodie (10% off with the code "WELCOME10"):
👉 https://bit.ly/bprp_merch

"Just Calculus" is dedicated to helping students who are taking precalculus, AP calculus, GCSE, A-Level, year 12 maths, college calculus, or high school calculus. Topics include functions, limits, indeterminate forms, derivatives, and their applications, integration techniques and their applications, separable differential equations, sequences, series convergence test, power series a lot more. Feel free to leave calculus questions in the comment section and subscribe for future videos 👉 https://bit.ly/just_calc
---------------------------------------------------------
Best wishes to you,
#justcalculus

geometric of geometric series equationdouble geometric seriesgeometric series equation